We’ve trained kids to know that math is a set of specific, discrete rules that, when followed, yield the correct answer.
They memorize math rules like:
- Whatever you add to one side, you must add to the other.
- FOIL
- Distribute
- PEMDAS
- Plug in 1 for x (then 0, then -1)
The rub is, that they don’t understand why the rules work. Which might not be a big deal – but the rules don’t always work!
We got to see The Math Rules in action.
For two days, in my #PowerMath class (officially Developmental Math – the class two levels below College Algebra), we’ve been working on this problem:
The goals were these:
- Practice and understand the order of operations.
- Compare this to working with variables.
- Learn to experiment with the math rules to discover how things work.
What I saw was mind-boggling. I knew the skill level was low. But I had no idea the blind following of math rules was so widespread and detrimental.
Goal #1: Practice and understand the order of operations.
When given the problem, and asked to coach me on various ways to do it, someone requested we use the order of operations and “do inside the parenthesis first.” So we did. They instructed me to write it like this:
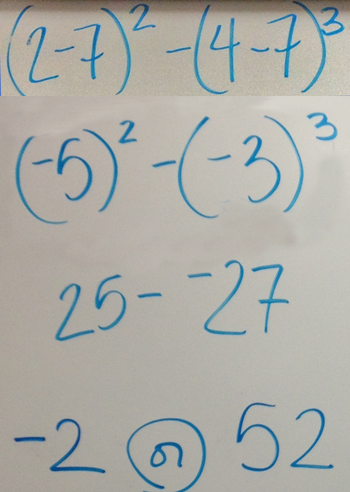
All was cool until the end when there was a bit of an argument on the final answer. So we listed them both as possibilities.
Although the act of doing the arithmetic inside the parenthesis is valid, we had much discussion on the positive and negative signs running around.
I had encouraged everyone to chime in with their own ways to find the answer, and someone suggested that I do the exponents first. They coached me to write this:

Alas, we had yet a different answer than the first two.
This is a common mistake. But I was hoping that through other experimentation they would see how we could fix this.
Someone else pointed out that we could FOIL. I asked them to explain exactly what they meant by it and they told me how to draw the arrows:
By the time we stopped, the class was in a full out #mathfight. Some students believed we needed to handle the exponents first, then do FOIL and others thought we should keep the exponents on the bits after we used FOIL. We decided to postpone this version until we worked out some of the others.
The use of FOIL here doesn’t work because the two binomials are being raised to a power. Furthermore, they are being subtracted, not added.
Goal #2: Compare this to working with variables.
The comparison of real numbers to variables has three main points:
- Variables represent numbers.
- Any math rules you can apply to numbers you can also apply to variables.
- Any math rules you end up using on your variables, must also work with numbers.
To help illustrate this, I removed the 7’s in the problem and replaced them with x’s. Our initial tries were interesting.
This experiment, as suggested by the students, applied the same “method” of squaring the inside pieces as one of the purely number versions above:
At which point, someone suggested that we subtract 4 from both sides.
Line #3 here is incorrect. Also, there is no “both sides” because there is no equals sign.
We went to another part of the board to start over and give it a try like this:
Here it was suggested that we set x=1.
Since x=7 (by the design of the problem) it doesn’t follow that x=1. I believe the student was remembering graphing an equation where x is the dependent variable. Also, note that the last line is merely a permutation of the symbols in the expression – it is not equivalent to the previous line.
Goal #3: Learn to experiment with the math rules to discover how things work.
We have a rule in class:
Until you decide differently, everyone is wrong. Even the teacher and textbook.
So I don’t tell them when the answer is right. The skills they are learning are the middle of problems they’ll be solving in the future. They’ll never get confirmation that what they are doing is right or wrong.
There’s no back-of-the-book solutions for the middle of a calculus problem.
Checking themselves, and having confidence in the answers that they have confirmed, are vital.
And yet they get highly frustrated with this.
Why? Because they’ve been trained that they should follow the rules and the teacher or back-of-the-book will tell them if they’re right.
And I’m refusing to play that game.
The full and complete math rules, that go with those above, are:
- Whatever you add to one side, you must add to the other, when you have an equation, not just an expression with no equals sign.
- FOIL when you are multiplying two sums, not subtracting them.
- Distribute exponents over products, not sums.
- PEMDAS works only when you know what each term really means and how to use them.
- Plug in 1 for x (then 0, then -1) when you are graphing an equation where x is the dependent variable.
But those last bits – the ones in bold-italics – those are ignored. They see an exponent outside of a set of parenthesis, they want to put the exponent on the inside pieces. They see two sets of parenthesis, they want to FOIL.
Thoughts? Share them in the comments and/or tweet them out:
Related articles
- Break the Rules and Get Fired!
- Numbers Instead of Variables
- PEMDAS and a Stupid Arbitrary Rule
- How to Create an Inquiry Zone for Math Learning

This post may contain affiliate links. When you use them, you support us so we can continue to provide free content!



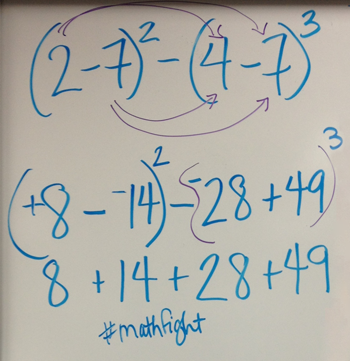

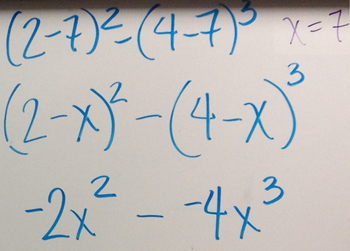





Well said, Bon! Rules without understanding are useless. As I read this, I was reminded of a recent experience subbing in an advanced 7th grade math class. We were working with equivalent fractions, and one student told me I needed to multiply the numerator and denominator by the same number. I asked why. He said, “Because you need to do the same thing to mama as to papa.” Huh? Again, I threw out the why question. Nobody knew. I explained it the same way I learned it – http://www.suedowning.blogspot.com/2011/01/equivalent-fractions.html. The response was very positive, and they asked why nobody ever told them that before. That was a question I couldn’t answer.
I just wonder how we got ourselves in this mess!
And how long will it take (and how much effort) to pull ourselves out?
So glad, though, there’s the internet. People like us can connect and start moving the revolution forward.
Thanks so much, Sue!
I do think that CGI math is a giant step in the right direction. I sincerely hope it is not too little, too late. There is a lot of ground to make up. http://www.wcer.wisc.edu/news/coverstories/cgi_math_encourages_ingenuity.php
Holy cow, Sue! I didn’t even know about that. Thanks so much!