In one of their recent podcast episodes, the Data Science Imposters discussed the prisoner’s dilemma. Here’s two takes on how to decide if you should confess or not. Continue Reading →
Response to Data Science Imposters’ Prisoner’s Dilemma
Tag Archives: puzzles
20 Challenging Puzzle Types
A list (and links) to 20 puzzle and riddle types you can try with your kids. Continue Reading →
Puzzles and Riddles that Improve Math Ability
Puzzles and Riddles improve math and logic skills. Here’s a list of good ones to try! Continue Reading →
Probability Tree Diagrams as Puzzles!
Probability tree diagrams are a tool to help students find probabilities. But they can also be a puzzle. Learn how and get the FREE DOWNLOAD here! Continue Reading →
5 Room House Puzzle
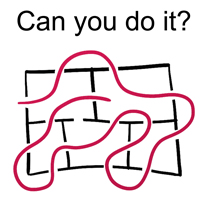
I worked on the 5 Room House Problem for more than 20 years. And yet I still assign it to students. It’s good for thinking and learning, and stating the problem. Continue Reading →
Personalized Number Puzzle Gift
Remember those email number puzzles that you get every now and then? Now’s your chance to make your own – easily! Continue Reading →
8 Digit Puzzle – Rules, Downloadable and Questions to Ponder
Have you tried the 8 Digit Puzzle? Here’re the rules, a downloadable copy and some fun questions to ponder about it! Continue Reading →
A Halloween Puzzle: Rubik's Cube
Can you figure out what’s missing in this Rubik’s Cube Halloween costume? Continue Reading →
Riddle: What are the ages of the kids?
Here’s a fun logic riddle to help your kids (or you) practice some logical thinking skills. Continue Reading →
Math Puzzle Inventing – Can You Do It?
Have you seen those email math games that return your birth-year? Think you can make your own? Continue Reading →