Someone showed me the 5 Room House Puzzle in the third grade. I worked on it a few hours every year until I was in graduate school. (Yes – a few hours every year!)
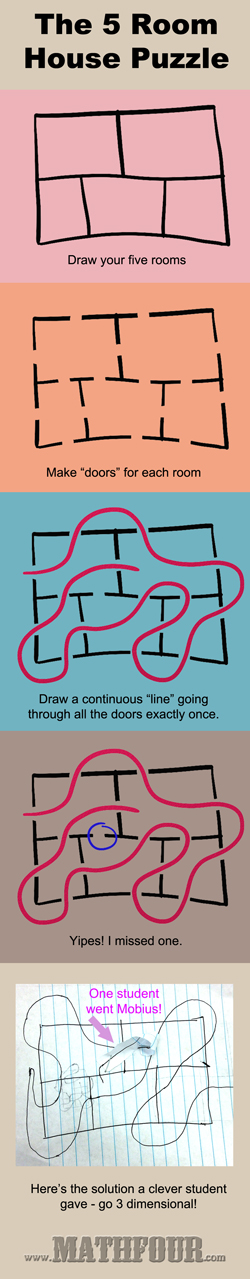Only after 20 years, did I realize its complexity. And that it’s impossible.
There was no internet back then. Or at least none that I had access to. So I was unable to discover the futility of my endeavor.
But is working on the 5 Room House Puzzle futile?
Thinking and learning include two things: knowing what the problem is, and struggling to figure out the solution.
The benefit of working on the 5 Room House Puzzle is that you learn, in your gut, how to state the problem.
Various questions on the way to stating the problem are:
- If I worked through this systematically, can I find the solution?
- Is there a different way to look at this?
- Is there some importance behind the five rooms?
- If I change the number of the rooms or the layout of the house, does that make it easier?
The original problem is never stated correctly.
In many explanations of how to solve word problems, you’ll see the statement, “Rewrite the problem in your own words.”
That’s because the question, as it’s written, isn’t always what it seems.
And this is the case with the 5 Room House Puzzle. The solution isn’t the path through the doors. Nor is it the fact that it’s impossible.
The solution is the answers to:
Why doesn’t it work? What would make it work? Am I convinced that it’s impossible, or am I just trusting the Internet?
Assign the 5 Room House Puzzle to your kids!
I will occasionally assign the puzzle to a group of students. I usually offer them a reward of one dollar to each person who can solve it.
If I offered them $1 million, they would be suspicious.
Sometimes I look at it as vengeance: “If I worked on this problem for 20 years, so should you.”
But mostly I know that if I ask the right questions, I’ll encourage thinking and learning.
How about you? Will you assign it?
Share your thoughts and experiences in the comments!
This post may contain affiliate links. When you use them, you support us so we can continue to provide free content!
Ms Crowder,
It may be of interest to you that this drawing (without spaces for the doors) was given as early as 1844 by T. Clausen as a different type of line puzzle. His challenge was to draw or retrace the original outline of the figure with the minimum number of restarts (pick up and move the pencil)
I have not been able to find when it took up the “5 rooms” approach of crossing each wall.
Also it was reported that Shongo children in the old Congo in Africa used to have contests drawing outlines like these and deciding which could and could not be a single path. Not sure how late this would have been, but probably early 20th century.
Hey Pat – thanks for that info!
Hey I solved it look at the middle line that touches the top it separates the right and left room not only down the middle but at the top too and can be entered that was as a line and doesn’t count against the right and left side as it is it’s own line. I would upload a pic but don’t know how
I have been working on this puzzle for 46 years and still trying.
Me too, Jose! (Well, only about 37 for me – but still a darn long time!)
My father showed this to my siblings and I over 40 years ago…I keep trying to no avail.
It’s a tough one, alright!
I think i have a real solution who do i contact
http://image.noelshack.com/fichiers/2015/03/1421549869-sans-titre.png
am i wrong
You missed the top right line segment. See here: https://www.evernote.com/l/ABVjWJ05hh9ANrlnUtc_dIRKrBOlAIL96kg
(Great try, though!)
My wife gave this to me and said a lawyer she works with has been trying it since 7th grade with no success…I, naturally, had to either do it or prove that is couldn’t be done.
Can’t be done.
The three bigger blocks have 5 sides, while the two smaller ones have 4.
This means 5 and 4 lines must be involved in those blocks to satisfy the goal.
The two smaller could either have lines go in, out, in, out (ioio) or oioi.
That works because no line is trapped inside or outside.
A five sided box, however, has to go either ioioi or oioio, which can not be sustained.
The total amount of lines has to be uneven:
2, 4 sides boxes plus 3, 5 sides boxes
(2)ioio + ioioi + (2)oioio
(2)(2i2o) + 3i2o + (2)(3i2o)
4i4o + 3i2o + 6i4o
13i12o
The ins and out don’t match so a line will always be separated from the goal.
Try with any number of 4 sides blocks and it is always possible, as it is with any even number of 5 bordered blocks.
The only way to win is to have a single line go through a joint (high would count as crossing 3 lines at the same time but that is, apparently, not allowed.
Actually, I don’t think it’s doable with multiple 5 sides boxes because it eventually/immediately excludes the lines from each other.
Indeed, JustMyke. It is impossible. Alas, a more formal proof of this exists. I believe it’s a Graph Theory thing, but I haven’t looked at it in years.
Thanks for stopping by!
This was given to us in 6th grade in our Apex group…..the only solution we ever came to was this.
The rules state one Continuous line that does not cross through the same wall twice…..so we drew a small 5 block puzzle (5 room house) and one continous line going from the top right corner to the bottom left or vice versa….the catch the line was ultra thick so that it covered the entire surface
Sneaky, but awesome!
If you’ve had a course in Graph theory, this isn’t a difficult question anymore. The problem can be seen as a graph of 6 vertices (rooms A, B, C, D and E, and the area F outside the rooms). Looking at the drawing, it is clear that the valency of vertex A is 5, which is an odd number. Then it’s immediately clear that this graph can’t be an Euler graph, so there doesn’t exist a circuit that passes all edges (the doors between the rooms).
Great point, Sander. Alas, I was in the third grade when I started on the problem. And it wasn’t until my graduate course in Graph Theory (my only one) that I learned this. It was a long time between 8 years old and grad school. #sigh
Thanks for stopping by! 🙂