We did an exploratory lesson on inequalities in the College and Intermediate Algebra classes last week. Here’s the reduced version (and the punchline) of that discovery process:

The process is slow.

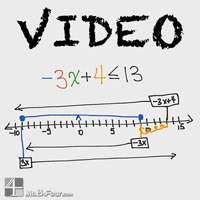
If you want to try this in your class, do it slowly. Start with .

The big jump is the next step. Ask them where is. They’ll all want to put it at 9. Remind them that you want where  as a team lives.

It’ll still be freaky, but some will get it.

Show how moving 4 to the left (or subtracting 4 from ) will put the familiar regular-all-by-itself x on 9.

Integrate in the 3 by giving them . Then play the same game: move left 4 then scale down 3.

And finally handle the “teeter-totter” of the negative sign by flipping the whole thing over.

It takes a while, but it’s worth it.

And don’t think it’ll be easy. They’ll fight the whole way. Remember, they’ve been trained to memorize steps, not think about what’s happening.

Try it and let me know what you think in the comments!

P.S. Pin this…

This post may contain affiliate links. When you use them, you support us so we can continue to provide free content!