I’m currently enrolled in a Stanford University MOOC (massive open online course) called How to Learn Math. It’s designed for parents and teachers, but it’s a variation of the face-to-face course they teach to students.
What most interested me today was the discussion on “compression.” And how I can apply this to teaching College Algebra.
Your brain compresses what it learns.
When you learn a new math concept, it takes it up a great deal of space in your brain. After you practice it and master it, it becomes compressed. Which means it sort of becomes second nature.
The “steps” you used to learned to do the process, you now can do in one step or even instantaneously.
As you learn more advanced things, those older processes don’t take you near as long as they used to. But the new things you’re learning do take a long time. At least until they get compressed.
Here’s what learning looks like when your brain compresses:
But if you don’t have a good sense of number, this compression process doesn’t work for math concepts. Which means your learning looks like this:
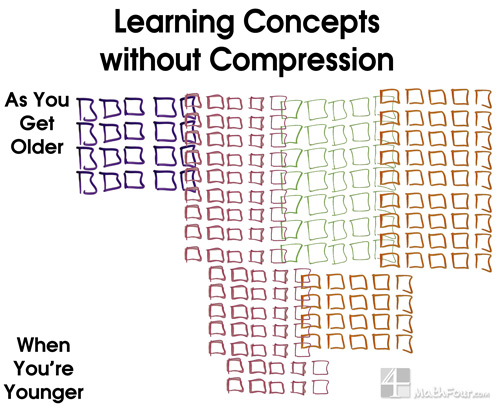
You’re doing every method – fully with all the steps – all the time!
And it happens!
I call this process “internalizing.” Once you do something many times and fully understand it, you internalize it. It just becomes part of who you are.
It never occurred to me that my students may be unable to internalize because of their lack of number sense!
Which means that every new process they learn gets crammed into a set of processes that they are holding onto – and they keep every single step!
What if we taught College Algebra differently?
At this level they’ve seen the algorithms – maybe even many times. There’s not a single person in the class that hasn’t been subjected to solving for x, PEMDAS, or graphing.
What they haven’t been able to experience is a true sense of number.
So I’m wondering if college algebra shouldn’t include a huge unit on number sense. Perhaps even 1/2 to 3/4 of the semester.
Can I teach College Algebra without variables?
Is it possible for adults to gain a sense of number and compress the algorithms they already know in 14 weeks?
Of course the algorithms will be reinforced by the arithmetic (after all – arithmetic is algebra). But what will that look like?
And should I try it?
Please let me know your thoughts in the comments, or via Twitter (tweet “Do it!” or “Don’t even try!“).
I’m itching for some feedback on this one!
This post may contain affiliate links. When you use them, you support us so we can continue to provide free content!









I’ve often thought the key to math is doing a lot of it until it becomes second nature. I tell my students to compare it to learning to walk. Watch a toddler and see how much effort it takes to learn, but you haven’t given it a thought in years.
How do you get that number sense? With our games we try to have lots of different activities of different types, so that students see the same concept over and over but in different situations.
However you do it, I agree, number sense is a foundation too many people do not have, and just like in construction, you can’t build very high without a foundation.
Thanks for your thoughts, AnnMaria.
Repetition is important, but only if the foundation gives you the ability to make it compress. Otherwise you’re just trying to be a computer running a gazillion subroutines!
Great illustration, Bon. The only thing I’d suggest is to replace is the “younger” vs “older” labels in your comparison with “novice” vs “expert”.
Have you read any of David Tall’s stuff about mathematical thinking (http://homepages.warwick.ac.uk/staff/David.Tall/themes/three-worlds.html) ? He says that as expertise in math develops, processes become encapuslated (aka: compressed) into what he calls “procepts” — “thinkable concepts” or hybrids of process + object which can be manipulated in their own right.
Working memory (WM) is known to have a very limited capacity (newer research has lowered this from 7 plus/minus 2 items to 3 plus/minus 1). Without encapsulization/compression, WM fills to capacity with the calculations and single steps which all must be juggled individually and sort of held in suspension there until they can be assembled into a problem solution.
When students have to deliberately recall and then ‘drive’ each little step in a more complex process, WM capacity is exceeded and cognitive overload ensues. Some of the little pieces may be displaced by other little pieces which need attention. Unable to recall how the individual steps contribute to the solution, the student may become lost inside the bigger process. Unable to ‘see’ a solution taking shape, the student may shut down.
Developing fluidity is essential, and facility in fractions especially is now seen a sort of bellwether of future success in math.
Very nicely articulated Sue!
I had no idea that working memory was so small. I was still back on the 7.
My brain just realized that it can’t take much more and is wanting to shut down. Time for coffee!