Factoring Polynomials – FREE Worksheet

In factoring polynomials it’s sometimes handy to break up the work graphically. Lisa Nuss, a member of Sam Shah’s New Blogger Initiation, shared a handy graphic organizer last week.

I struggle with factoring polynomials, myself, so I wanted to give it a try.

It started out easy.

I started factoring polynomials with 1 as the leading coefficient (i.e. x² has no messy number in front of it).

I factored:

First, I put the first and last terms in the boxes. (Note that this graphic organizer works the same way as a multiplication table.)

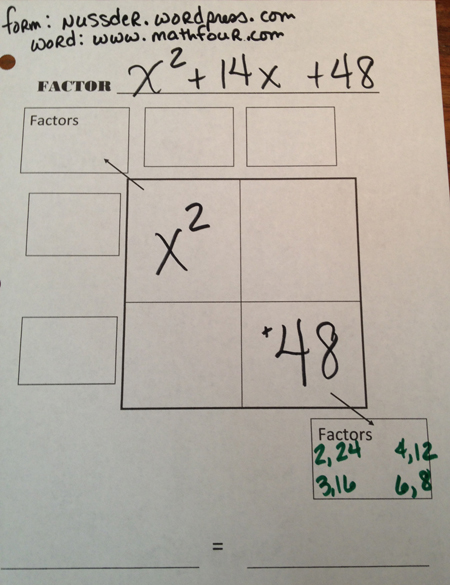
Then I factored those two in the given “factors” boxes. I determined which factors of 48 would add up to 14, and filled in the chart appropriately.

I was done with that factorization.

Yay me!

Then I got into harder stuff.

Lisa put in an extra “Factors” box to handle non-unit leading coefficients. So I went for a big dog:

Here’s how far I got before the breaks squealed:

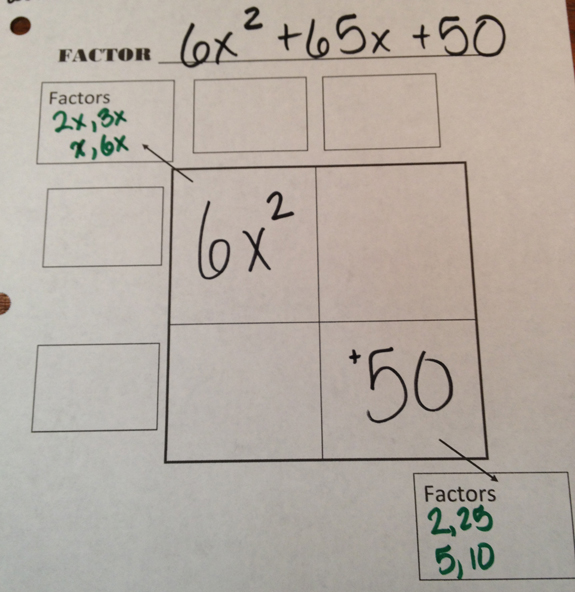
Factoring polynomials like this one shouldn’t be too much of a problem. Especially if you use a page protector and a dry erase pen to do the trial and error work, as Lisa suggested.

But I don’t have such fancy technology.

And I don’t like to erase my work. I want to see everything I’ve tried. For me, it’s very likely that I make a mistake and have to go back. And it’s a real pain to have to re-create everything.

So instead of playing trial and error with the one big sheet of paper, I created a Factor Trial & Error Boxes worksheet (or in Lisa’s terminology: a graphic organizer).

Here’s what my work looked like:

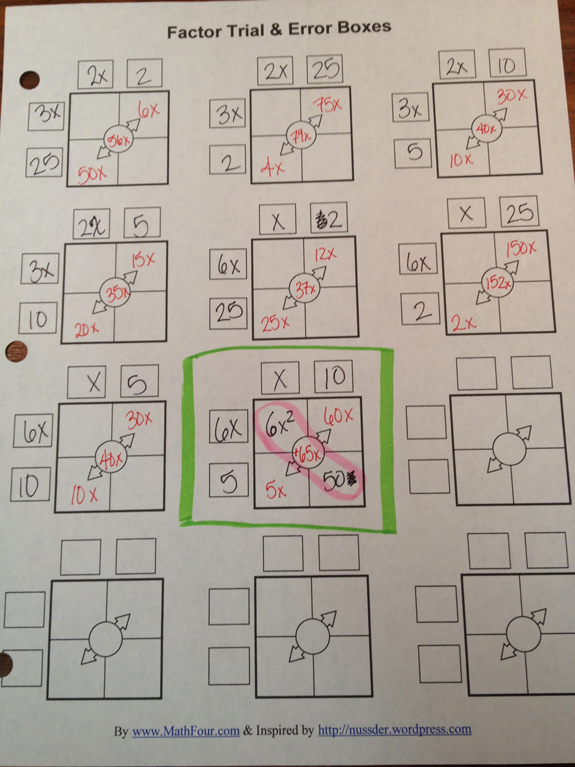
(It was a coincidence that the right answer was last, by the way.)

With that info, I could go back to my big graphic organizer and finish the problem.

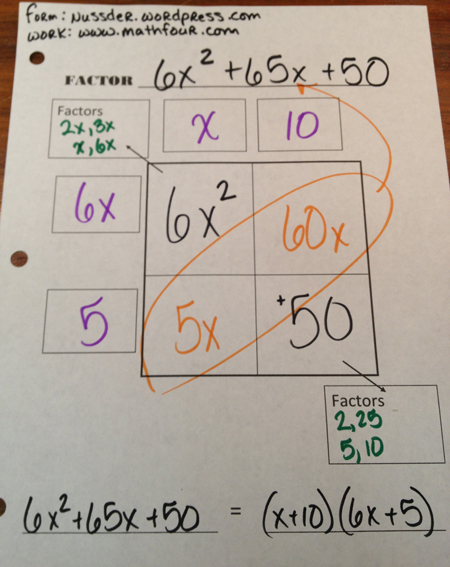
This was very pleasing. I was able to use Lisa’s graphic organizer and mine to make sure I didn’t lose any options in my my trial & error.

Will it work for you and your kids? Download the Factor Trial & Error Boxes worksheet now and give it a shot!

then

You might also like:

Compared to others on the market, best price and quality. ! Each and every of the products available through our website has been produced using the best base materials.

This post may contain affiliate links. When you use them, you support us so we can continue to provide free content!

11 Responses to Factoring Polynomials – FREE Worksheet

Colin Beveridge says:

September 6, 2012 at 5:06 pm

Oo, pick me, pick me!

There’s a quick way to find the missing magic numbers — find the factors of (ac) that add up to (b) — so for 6x^2 + 65x + 50, you’d look at 300; the possibilities are 1 and 300, 2 and 150, 3 and 100, 4 and 75, 5 and 60 (which works), 6 and 50, 10 and 30, 12 and 25, or 15 and 20.

It’s a little bit more work up front, but it saves you doing several grids.

- Bon says:
  
  November 7, 2012 at 7:03 am
  
  I think that may be the point, Colin. That method totally works for you (I’m guessing) – but it sends me into panic mode.
  
  My major prof in grad school trained me to “get my hands dirty” (he’d tell me that ALL THE TIME). So now I write every single detail out.
  
  And for our students – whatever works for them, should be what they use.
  
  Thanks for stopping by!
  
  - Charlie says:
    
    March 23, 2014 at 7:33 pm
    
    I had the same issue with many of my students trying to find the factor with a leading coefficient greater than 1. I found the umbrella method works great. Youtube it and it works every time. The kids with the most trouble like this one the best because they can’t get it wrong if combined with the box method.
    
    - Bon says:
      
      April 9, 2014 at 4:41 am
      
      Thanks, Charlie. I found it here: http://youtu.be/FxTiogyhwfc?t=48s I find the details the guy uses a little cumbersome. It took me a while to see that his “find the common factor” on the box method was fancy math words for just figuring out what to put on the left and top.
      
      However, I see lots of value in making an umbrella on top of the trinomial to help students focus on the important pieces to put in the box!
      
      Thanks so much for sharing!
      
Cristina says:

September 11, 2012 at 5:02 pm

This is an interetsing method… I didn’t come accros it before… I like it! Do share more 🙂

- Bon says:
  
  September 14, 2012 at 3:28 am
  
  Thanks, Cristina! I’ll happily pass along things as I find them. 🙂
  
Sara says:

December 9, 2013 at 2:19 am

Wow! So happy I found this! I’ve been teaching elementary and middle school for about 12 years. Now I’ve added high school math into the mix. Just recently acquired 4 Pre-cal students which I’m enjoying teaching! The challenge is mostly the varied math backgrounds of the students! Factoring polynomials will be our first review/re-teach workshop!

Thanks so much and wish me luck!

- Bon says:
  
  December 9, 2013 at 6:10 am
  
  How fun, Sara! Good luck and keep us posted on how it goes!
  
V Deck says:

March 2, 2015 at 7:55 pm

This is a nightmare for algebra 2 teachers when 8th grade and algebra 1 teachers teach kids this method. I’ve taught several student groups multiple years in a row, and the students I taught this or the “split the middle method” did not remember how to factor the next year, but those who I taught the old fashioned “guess and check” method remembered from year to year.

- Bon says:
  
  April 14, 2015 at 5:01 am
  
  I can totally see that. Once students learn to get their hands dirty, they can always go back and dig around to find the answer.
  
  Thanks for stopping in to join the conversation!
  
Tanuja says:

October 10, 2016 at 11:51 am

Thanks for sharing this! I’m like you, factoring polynomials like these gives me sweaty hands! The graphic organizer helps too!