There’s only a handful of actors in “math world.” Depending on the show, they play different parts: inverses, identities, etc. Continue Reading →
The Math Show Stars are All the Same
Tag Archives: algebra
2 Ways to Do Math Problems
Many math problems boil down to being a choice between two things. Why not try them both out? Continue Reading →
Inequalities on a Number Line
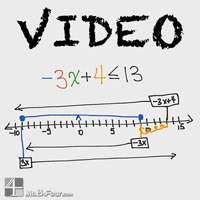
Y’know those weird rules on solving inequalities? How about explaining it using a number line? Continue Reading →
A Different Way to Teach College Algebra
If your sense of number doesn’t get established, your brain can’t compress math learning. I’m thinking about using this principle to teach college algebra. What do you think? Continue Reading →
Why People Aren’t Equal
What does it mean for people to be equal? And why are we using a math concept for people anyhow? Continue Reading →
Linear Programming Problems – How to Set Them Up
Do your students struggle with setting up linear programming problems? Do you? Here’s a handy list of steps that will help. Continue Reading →
How to Teach Square Roots
Did you know you can teach square roots with fractional exponents – and it just might work better that using radicals! Continue Reading →
Fahrenheit to Celsius – Graphically!
The conversion from Celsius to Fahrenheit and back again can be strange. One way to understand it is on a graph. And you can use this to teach some linear algebra too! Continue Reading →
Teaching Square Roots with a Non-PC Analogy
What do you think about this analogy I use when teaching square roots and other manipulations of radicals? It’s pretty non-PC, but it seems to work. Continue Reading →
How to Introduce Function Transformations
Do you teach transformations? Are they tough to introduce? Try this activity! Continue Reading →