
Turn a dry factor tree into a beautiful fall math craft project! Continue Reading →

Turn a dry factor tree into a beautiful fall math craft project! Continue Reading →
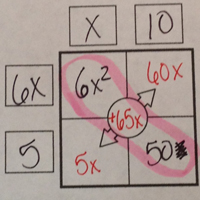
When factoring polynomials it’s sometimes nice to use a graphic organizer to keep track of all your work. Here’s a free one! Continue Reading →

A totally fun video on factoring polynomials along with a worksheet for your kids and a super parent-teacher cheat sheet for you! Continue Reading →

A pseudo review of a book I’ve wanted for a while. You Can Count on Monsters by Richard Even Schwartz illustrates the numbers from 1 to 100 – with monsters and factorizations! Continue Reading →
Alright, you might not win friends with this, but the more you can do arithmetic in your head… well… okay, you can’t influence people with it either. Regardless, it’s handy to know and helps with multiplication. Yesterday I posted a couple of videos about factoring numbers into primes. Now it’s time to put those to work.… Continue Reading →
Factoring is traditionally considered an “f-word” for students of math. But it really has its good sides. First, here’s two ways to do it with regular numbers. Continue Reading →
Can you quickly tell if a number is divisible by 3 or by 9? With this trick you can impress everyone – well, not EVERYone, but a few anyhow. Continue Reading →
We learn to factor polynomials so we can solve for x. But some things aren’t so easy to factor (most things?). And some things are just down right a pain in the bottom. Here’s a way to never have to do it again! Continue Reading →