This is Day 21 of 31 Days of Math Learning Success. Follow all the days here and check out others that are writing for 31 days here.

Ultimately, you have to pursue your own path, not someone’s idea of the right path.
~Baz Luhrmann

Take the following True/False quiz about your favorite “math person.”

1. True or False: They have never been wrong on a math problem.
2. True or False: They made all A’s in math.
3. True or False: They never failed a math test.
4. True or False: They understood everything the math teacher said.
5. True or False: They were never confused about a math concept.

Give yourself 1 point for each “true” and 0 points for each “false.”

If your score is 0, awesome. Skip to the next section.

If your score is 1-10. Read on.

Shattering the Myth

1. They have never been wrong on a math problem. FALSE Everyone who’s ever done math has gotten a problem wrong. You can’t learn math unless you mess it up, think about it and learn from it.
2. They made all A’s in math. FALSE Call your math friend. I’ll bet you a shiny donut that they have made far less than A on many many things in math. (I made an F in statistics!)
3. They never failed a math test. PROBABLY FALSE If you ask your friend about this one, he probably doesn’t even remember. He’ll say, “Well, I probably did. I just don’t remember many math tests.” He says this because he has a growth mindset. He used the grade he made on each test as an indicator of what to focus on for future learning.
4. They understood everything the math teacher said. FALSE People have at least 12 math teachers in their lifetime. If you have a friend in a math related field, she probably had 15-30. Which means she had gazillions of minutes of in-class time. Lot’s of time to lose train of thought. Lot’s of time to be lost.
5. They were never confused about a math concept. FALSE Every “math person” has been confused. Most have been so confused that they either skipped a concept (and hoped that later down the road they’d figure it out). Or they made up their own workaround to fake it.

Creating a Workaround

My favorite workaround was from a fellow teacher I knew long ago. He couldn’t remember the rules of addition/subtraction for integers. So he did this:

   

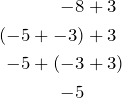
He would bust up the “bigger” integer into two pieces (what the new math calls decompose) and then partner the two pieces that were the same (except for the sign) and let them make zero.

Working around Subtraction

I’m developing some courses for parents about the new math. I’m amused that the popular way of teaching subtraction now is the way I used as a workaround back then!

I had no idea what was really happening in borrowing. So borrowing “from the guy next door” was weird.

But when they started teaching us how to double borrow, or borrow from zero, I just fell apart. So I created reverse addition for myself.

Turns out it was a pretty good idea.

Learning about Learners

Now it’s your turn. Go out and ask some people. Start with your “math friends.”

Ask them about the T/F statements above. Find out what workarounds they created.

And then create your own.

What math concepts are crazy hard for you? Can you figure out a better way? Do it.

You just might surprise yourself!

Are you enjoying the 31 Days of Math Learning Success? Share it on Twitter, Facebook and Pinterest!

This post may contain affiliate links. When you use them, you support us so we can continue to provide free content!