Part of Wordless Wednesday…
The beautiful and talented Heather at Freebies4Mom.com sent me a post on An Easy Way to Convert Celsius to Fahrenheit.
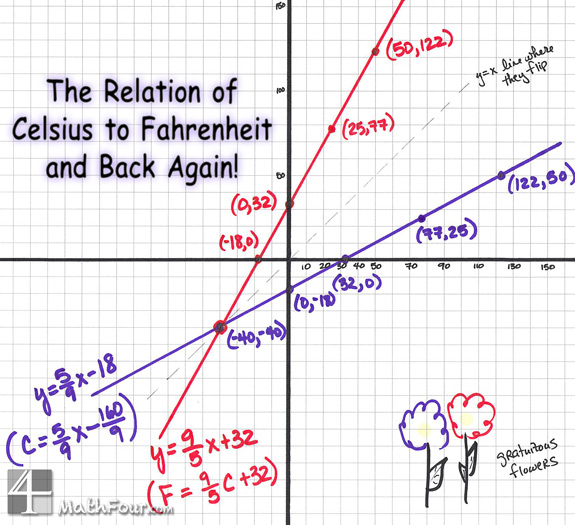
It inspired me to draw the graphs of Celsius in terms of Fahrenheit and Fahrenheit in terms of Celsius.
Notice these two intersect at (-40, -40). Which means that -40°F is -40°C!
Use it to convert temperatures.
The x values on the red line are Celsius – so find the °C you have and then look at the y-value to convert to °F.
It’s just the opposite on the purple line.
Okay, fine. This isn’t the greatest way to convert – but it’s exciting to see it graphically. And it might be easier to convert this way for someone who’s more visual.
Use it to teach math!
These two lines are inverses of each other. So the coordinates of one are switched to make the other.
Also, they mirror image across that 45° line. I marked the line with dashes and wrote ![]() on it.
on it.
And if you’re into this, their functional composition (both ways) is… x! (not factorial)
Compare and Contrast…
Take a look at the way J.D.Roth did it and then look at the graphs I have. Let your students find the way they like the best. And encourage them to create new ways!
Oh, yeah – and share what happens in the comments!
This post may contain affiliate links. When you use them, you support us so we can continue to provide free content!


Cool
Thanks, Random Guy!
what i’m trying to noodle through is 1) why do they mirror each other at 45 degrees x=y and 2) why do they converge at about -40 degrees? why that point rather than zero? Can you help?
The explanation I heard was this. Each system was based on the slope between two ‘arbitrary’ points.
For Celsius those points are the temperatures when fresh water freezes (0 C) and boils (100 C).
For Fahrenheit the points were way more arbitrary. That is the freezing point of sea water (0 degees) and body temp, which was thought to be 100 degrees at that time.
The slope of each system determines the convergence at -40 degrees and nothing more. Any two non-parallel lines will meet somewhere and this is where they meet.
I hope that is useful.