Check out this photo of two books explaining the addition of fractions – one from 1880 and one from 2009. Continue Reading →
Adding Fractions – Then & Now
Tag Archives: adding fractions
Adding Fractions with Cuisenaire Rods
Christine asked me via twitter for some resources for Cuisenaire rods and teaching fractions. Of course I can’t pass the opportunity up to do some fun things with my rods! Continue Reading →
Why It's Okay to Add Denominators when Adding Fractions
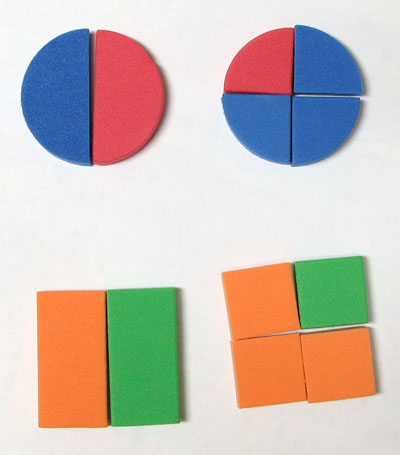
So you think you HAVE to find a common denominator when adding fractions? Well, not really. Especially if you want to inspire kids to really think like a mathematician and do some inventing! Continue Reading →
Practicing Math Skills Early in Life Is a Brain Changing Event
New research by Stanford Medical’s Dr. Vinod Menon shows that children can develop math skills and cognition early and quickly – with differences shown in as little as one year! Here is a way to start math learning early. Continue Reading →
6 Confusing Terms in Mathematics
Here’s how to understand and explain some of the top confusing terms in mathematics – pain free. Continue Reading →
Adding Fractions: Why Avoiding Common Denominators Works
I wrote this post showing that adding fractions can be done using the algebraic definition of addition of rationals: MathHeadInc (via twitter) has requested a video showing why this works. I aim to please. Here is the video showing why adding fractions using a common denominator is the same as the definition of addition of… Continue Reading →
How to Never Find a Common Denominator Again
Do you keep struggling to teach common denominators? Do your kids just not “get it”? Well, it’s time to quit. Yep! You can teach (and do) fractions without ever finding a common denominator. The key is in the definition of addition for rational numbers. If you have two numbers and the sum of them is . Granted… Continue Reading →